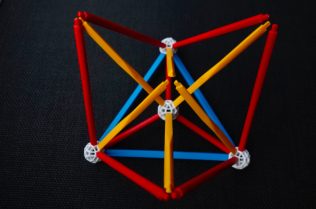
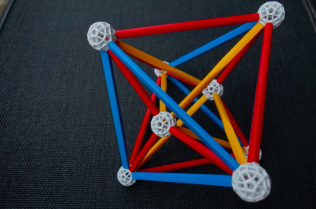
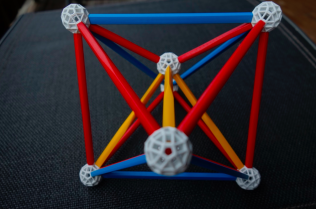
ZometooL MODEL OF A 16 CELL
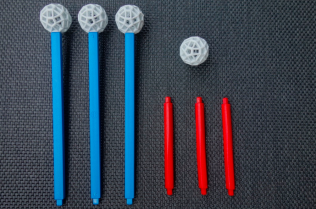
Here we describe how to build a projection of the 16 cell, one of the six regular polytopes in 4-dimensional space, using the zometool system. For the model you need
- 8 balls,
- 6 long blue (b2) struts,
- 6 long red (r2) struts,
- 6 medium red (r1) struts,
- 6 long yellow (y2) struts.
Of course one can scale down the model to b1-r1-r0-y1, but this becomes quite tiny.
For further information and instructions you might also wish to consult one of the following webpages:
- Eusebeia 4d visualization,
- David Richter's webpage about the Zometool models for the 16 cell,
- David Richter's webpage about the Zometool Triality.
The pictures below were taken by Eva-Maria Gassner.
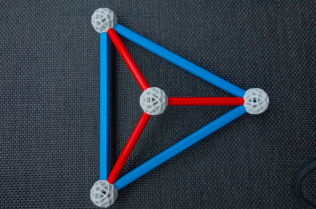
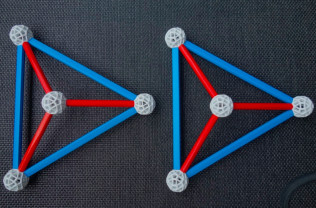
Step 1
Use the blue and the medium red struts to build two regular triangles with small red pyramids mounted on top.
This Zometool model of the 16 cell shows all of the 16 tetrahedral cells, but they appear differently squashed as a result of the projection. Moreover, in contrast to the vertex-first projection all of the 8 vertices and all of the 24 edges are visible in this projection.
This model is connected to the other two models (see here and here) by the Zometool Triality.