A ZOMETOOL MODEL OF A 16 CELL
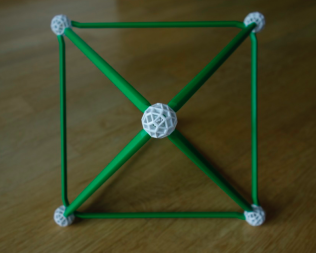
Here we describe how to build a vertex-first projection of the 16 cell, one of the six regular polytopes in 4-dimensional space, using the zometool system. For the model you need
- 7 balls,
- 6 long blue (b2) struts,
- 12 long green (g2) struts.
Of course one can scale down the model to b1-g1 (medium size) or to b0-g0 (mini version).
For further information and instructions you might also wish to consult one of the following webpages:
The pictures below were taken by Eva-Maria Gassner.
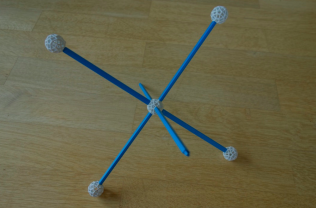
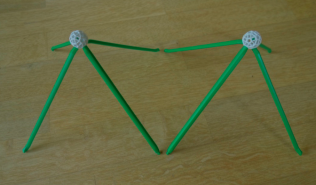
Step 1
Use the blue struts to build the 'axis' of a rectangular coordinate system. Put balls on all but two opposite ends. The ball at origin of the coordinate system is the central vertex of the projection.
Step 2
Use 4 of the green struts to connect the 4 balls at the ends of the blue struts.
Step 3
Use the remaining green struts to build two pyramids with a square base.
Step 4
Mount the two pyramids on the two remaining loose ends of the blue coordinate system to complete the model.
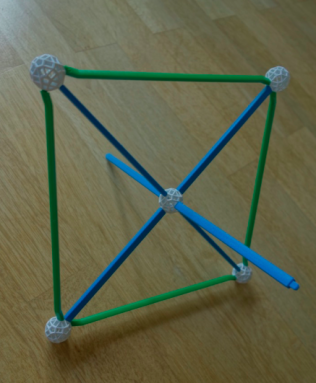
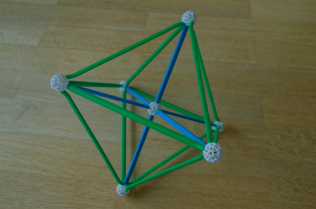
The model shows the central vertex surrounded by 8 tetrahedral cells. The remaining 8 cells are visible as triangles on the boundary of the model, since they are viewed under a 90 degree angle. In this way, all 16 cell of the 4d polyhedron are visible. Note that under the projection the tetrahedral cells (which are indeed regular tetrahedra in 4d) have become orthoschemes. Also note that only 7 of the 8 vertices are visible. This is because the central vertex is the image of